Measurement of the W Boson Helicity in Top Quark Decays
by the D0 Collaboration
December 11, 2007
One of the goals of particle physics is
to determine what fundamental particles exist in nature, and the rules
for how they interact with one another. Some, like the electron,
have been known for over a century, but we've continued to find new
ones since then. One of the most recent additions was the top
quark, discovered at Fermilab in 1995 and still the heaviest known
fundamental particle. The
current theory of particle physics, the "Standard Model",
had predicted that this quark must exist, but even so its large mass
came as a surprise, and raises the possibility that the top quark may
show the effects of new physics at energies that we've not yet
directly explored.
In addition to predicting the top quark's existence, the Standard Model specifies many of the properties of the top quark. In particular, we know the "rules" a top quark follows when it decays -- that is, when it transforms into lighter particles. We expect the top quark to decay to two particles, a W boson and a b quark. What's more, we can predict detailed features of these particles, even down to something as seemingly esoteric as the helicity of the W boson.
Helicity is the relationship between the direction in which the particle is moving and the "spin" of the particle. Spin can be considered as a directional arrow that's a feature of the particle itself, not of the particle's motion. For a given type of particle, the length of this arrow is fixed (we say, for example, that top quarks have "spin one-half" and W boson have "spin one"), but the direction of the arrow can vary from one particle to another. Helicity is the relative direction between the spin arrow and the arrow representing the particle's motion. For W bosons, there are three possibilities (spin arrows are red and purple, arrows representing motion are blue and green):

As the above diagram suggests, we expect that 70% of the W bosons will have zero helicity, 30% will have negative helicity, and almost none will have positive helicity. If we were to measure anything else (for example, if we find a large number of W boson with positive helicity), we would know that the Standard Model is incorrect -- and that would be exciting. Our hope is always to expand our knowledge, and finding an unexpected result is often a good starting point.
Our measurement uses a sample of data collected by the D0 experiment at Fermi National Accelerator Laboratory from 2002 to 2006. To make the measurement, we need to do two things: first, pick out the small number of top quarks in our data from the vast swarm of other particles, and second, distinguish the various W boson helicities from one another. For the first step, we're helped by the fact that top quarks look quite different from most of the other stuff produced in the experiment. After (years of) improving our selection techniques we've reached a point where we can pick out top quarks pretty well.
For the second step, we use a variable known as &theta*, which is illustrated from the vantage point of an observer travelling alongside the W boson:

In addition to predicting the top quark's existence, the Standard Model specifies many of the properties of the top quark. In particular, we know the "rules" a top quark follows when it decays -- that is, when it transforms into lighter particles. We expect the top quark to decay to two particles, a W boson and a b quark. What's more, we can predict detailed features of these particles, even down to something as seemingly esoteric as the helicity of the W boson.
Helicity is the relationship between the direction in which the particle is moving and the "spin" of the particle. Spin can be considered as a directional arrow that's a feature of the particle itself, not of the particle's motion. For a given type of particle, the length of this arrow is fixed (we say, for example, that top quarks have "spin one-half" and W boson have "spin one"), but the direction of the arrow can vary from one particle to another. Helicity is the relative direction between the spin arrow and the arrow representing the particle's motion. For W bosons, there are three possibilities (spin arrows are red and purple, arrows representing motion are blue and green):

As the above diagram suggests, we expect that 70% of the W bosons will have zero helicity, 30% will have negative helicity, and almost none will have positive helicity. If we were to measure anything else (for example, if we find a large number of W boson with positive helicity), we would know that the Standard Model is incorrect -- and that would be exciting. Our hope is always to expand our knowledge, and finding an unexpected result is often a good starting point.
Our measurement uses a sample of data collected by the D0 experiment at Fermi National Accelerator Laboratory from 2002 to 2006. To make the measurement, we need to do two things: first, pick out the small number of top quarks in our data from the vast swarm of other particles, and second, distinguish the various W boson helicities from one another. For the first step, we're helped by the fact that top quarks look quite different from most of the other stuff produced in the experiment. After (years of) improving our selection techniques we've reached a point where we can pick out top quarks pretty well.
For the second step, we use a variable known as &theta*, which is illustrated from the vantage point of an observer travelling alongside the W boson:

We know what the relative probability of seeing various values of &theta* for different W boson helicities (&theta*
tends to be small for positive helicity W bosons, large for negative
helicity W bosons, and around 90 degrees for zero helicity W bosons). For technical reasons, the measurement is
easier if we take the cosine of &theta*. All we need to do is compare the values of cos&theta* in our data sample to the expected distributions. The results, for a set of data corresponding to one of the signatures the top quark can leave in our detector, are shown below:
In this plot, our data is shown by the points with error bars. The red line represents what we'd see if all the W bosons had negative helicity, the green line shows the case where they all have zero helicity, and the blue line shows the case where they all have positive helicity. The shaded area is the estimated background (meaning events that don't really have top quarks in them but still sneak into our sample). Numerically, we can say that the fractions of W bosons with positive and zero helicities is:
Fraction with zero helicity: f0 = 0.425 +/- 0.195
Fraction with positive helicity: f+ = 0.119 +/- 0.104
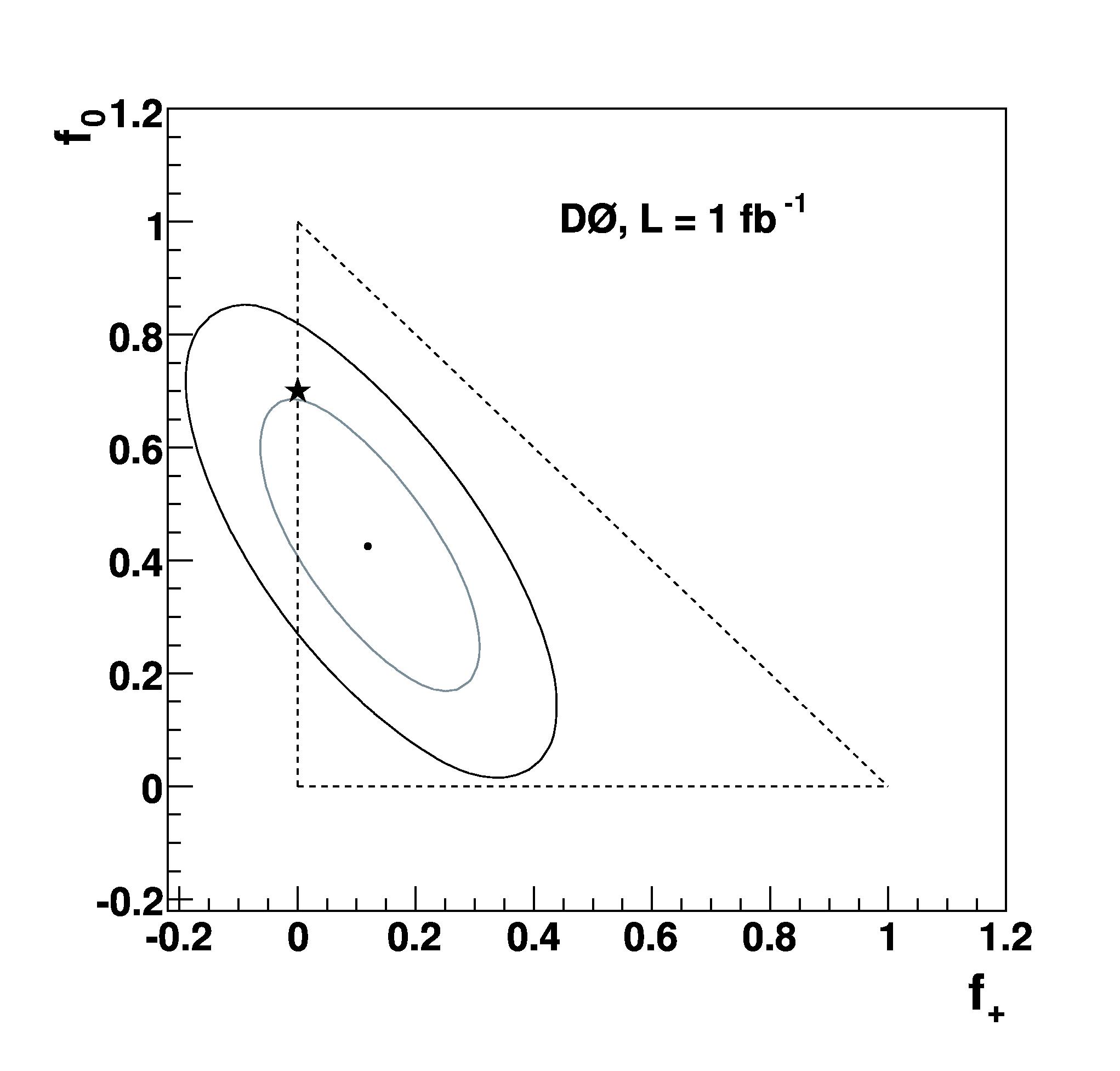
Remember that we expected f0 to be 0.7, and f+ to be very close to zero. So we don't find exactly what we expected. To see whether we can claim that this means there's physics beyond the Standard Model, we need to assess the probability of our result happening just by random chance if the Standard Model is correct. Graphically, the result looks like:
The star shows the Standard Model value, and our measurement is the dot (the triangle represents the limit of all "reasonable" measurements in which none of the helicity fractions are negative). The bigger of the two ellipses is the 95% confidence interval, meaning that if we were to repeat the experiment many times, we'd find an answer outside that ellipse 5% of the time. Since the Standard Model value is well within this ellipse, our result certainly cannot be used to claim that the Standard Model is incomplete. In other words, with the current sample size, it's not really unlikely that the discrepancy comes about just due to chance -- but we will look again when more data is available. The top quark may yet hold surprises for us!
More information about this measurement is available in our full article on the measurement.
This article was submitted to Physical Review Letters on October 31, 2007.
easier if we take the cosine of &theta*. All we need to do is compare the values of cos&theta* in our data sample to the expected distributions. The results, for a set of data corresponding to one of the signatures the top quark can leave in our detector, are shown below:
 |
In this plot, our data is shown by the points with error bars. The red line represents what we'd see if all the W bosons had negative helicity, the green line shows the case where they all have zero helicity, and the blue line shows the case where they all have positive helicity. The shaded area is the estimated background (meaning events that don't really have top quarks in them but still sneak into our sample). Numerically, we can say that the fractions of W bosons with positive and zero helicities is:
Fraction with zero helicity: f0 = 0.425 +/- 0.195
Fraction with positive helicity: f+ = 0.119 +/- 0.104
Remember that we expected f0 to be 0.7, and f+ to be very close to zero. So we don't find exactly what we expected. To see whether we can claim that this means there's physics beyond the Standard Model, we need to assess the probability of our result happening just by random chance if the Standard Model is correct. Graphically, the result looks like:
 |
The star shows the Standard Model value, and our measurement is the dot (the triangle represents the limit of all "reasonable" measurements in which none of the helicity fractions are negative). The bigger of the two ellipses is the 95% confidence interval, meaning that if we were to repeat the experiment many times, we'd find an answer outside that ellipse 5% of the time. Since the Standard Model value is well within this ellipse, our result certainly cannot be used to claim that the Standard Model is incomplete. In other words, with the current sample size, it's not really unlikely that the discrepancy comes about just due to chance -- but we will look again when more data is available. The top quark may yet hold surprises for us!
More information about this measurement is available in our full article on the measurement.
This article was submitted to Physical Review Letters on October 31, 2007.