We know that the world is made of particles: leptons and quarks. Leptons are electron, muon, tau and their neutrinos; quarks are six and in increasing order of mass are: up, down, strange, charm, bottom and top. We also know that the particles interact exchanging other particles, called gauge bosons. The Standard Model is the theory that describes the interactions among particles. An interesting feature of the quarks is that they are never observed isolated, they make hadrons, there are groups of two and three quarks, called mesons and baryons respectively. If a quark is created in a collision of protons and antiproton then this quark picks up other quarks to form a hadron, this process is called hadronization.

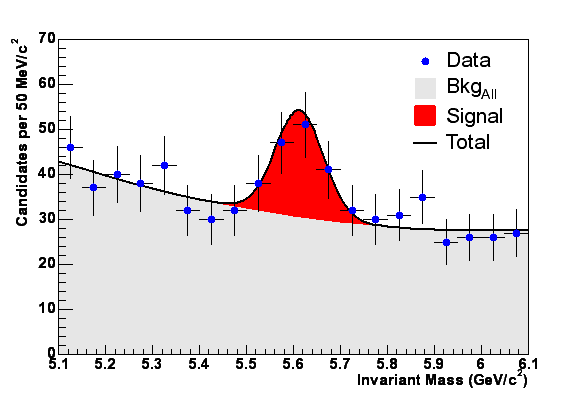In collisions of protons and antiprotons in the Tevatron, hadrons containing the quark bottom are produced, but those hadrons live less than 1/10¹² seconds. They become other particles due to the weak interaction. There are various models that try to explain how these hadrons decay into other particles. The most simple model predicts the same lifetime for all hadrons containing the bottom quark, however there are experiments that measured different lifetimes for the different B hadrons. Newer and more complex models have predicted different lifetimes of B hadrons very much in agreement with the measurements. One piece of this picture however is still not right, the measured lifetime of the Λ_b baryon does not agree with the theoretical prediction. Therefore a new measurement in a different environment can shed some light in this problem.

The lifetime of the Λ_b was previously measured in cases where the Λ_b became a set of particles that included a muon. In D0 we can measure that lifetime when the Λ_b becomes a J/psi and a Λ⁰. The advantage of this measurement over the previous ones is that all particles that come from the Λ_b are observed while in those previous measurements one of the decay products was missing.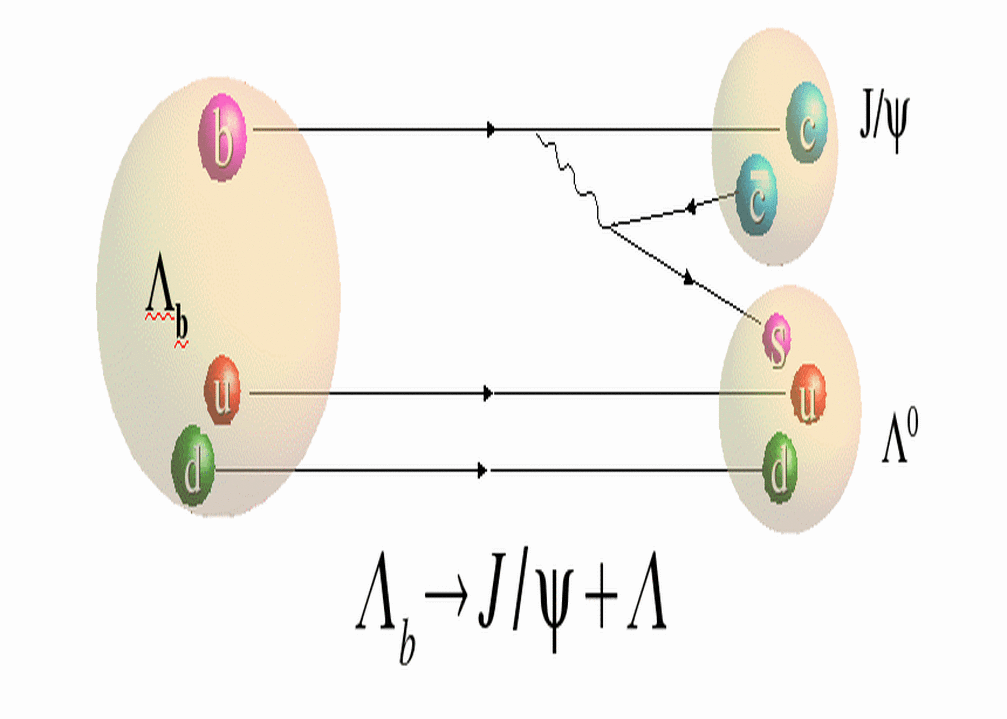

The measure of the lifetime of a particle means to measure the distance between two points, the point where the particle is created and the point where the particle ceases to exist and becomes other particles. In the case of the Λ_b this distance is of the order of hundreds of microns. To be able to measure these distances one has to have tools as precise as tens of microns, which we have. The D0 detector consists among other things of a component that is made of silicon that is capable of measuring distances of the order of hundreds of microns and therefore ideal to measure lifetimes of B hadrons.

If we had a set of Λ_b baryons we would be ready to make the measurement, however, every time a proton and antiproton collide in the Tevatron many things can happen, rarely a Λ_b will be produced. That is why we need to find a way to distinguish events that look like a Λ_b. We collect only those events that have the signature of a Λ_b, i.e., Λ_b becomes a J/psi and a Λ⁰, but the J/psi becomes two muons and the Λ⁰ becomes a proton and a pion. These particles are stable and can be seen in our detector.

Besides the Λ_b there are other processes that have the same signature and are impossible to separate. They are present in the data analyzed to measure the lifetime. Nevertheless they can be taken into account in the model used to measure the lifetime and in the end are not a problem.

Besides the Λ_b, the B⁰ meson can decay into a J/psi and a K⁰_s, having a very similar topology to the Λ_b decay used in the measurement. The lifetime of the B₀ was also measured using the previous decay. Theory predicts the ratio of the Λ_b and B⁰ lifetimes and therefore both measurements, the Λ_b and B⁰ lifetimes, can be used to estimate the ratio and compare with the prediction. Predictions of recent models that try to describe the decay of these B hadrons agree with the result of our measurements, validating their model.

A description of how we measured the Λ_b and B⁰ lifetimes is being published in the Scientific Journal Physical Review Letters.

Comments or questions to About the Λ_b lifetime analysis